The Schillinger/Berklee Connection

by Ted Pease '66

Joseph Schillinger (1895-1943), a Russian-born mathematician, music theorist, composer, and teacher, is a figure who has, unfortunately, become just a footnote in the annals of music history. However, in the early decades of the twentieth century, his influence on high-profile figures in American music was widely known. Schillinger developed a unique mathematical system of music composition and analysis and taught it to such musicians as Tommy Dorsey, George Gershwin, Benny Goodman, Oscar Levant, Glenn Miller and others. Miller apparently wrote "Moonlight Serenade" as a Schillinger lesson assignment. Gershwin used Schillinger's principles in the composing and orchestration of his famous opera Porgy and Bess.

Berklee founder Lawrence Berk was also among the students of Schillinger and was one of only 12 instructors that Schillinger authorized to teach his musical system. Berk studied with Schillinger while he was working as a composer and arranger for CBS and NBC radio in New York in the 1930s.

President Lee Eliot Berk recently came across 11 loose-leaf notebooks filled with Schillinger's teachings and exercises in his father Lawrence Berk's unmistakable handwriting. The binders bear the "Schillinger House" logo. (Before becoming known as Berklee, Lawrence Berk's fledgling school was called Schillinger House.) It has not been determined whether the material in the notebooks was written during or after his Schillinger studies or if Berk compiled the books in the 1940s as lesson plans for the Schillinger theory classes that he taught.

President Berk asked me to look through these notebooks to see if they might reveal anything about Berklee's early curriculum. Approaching the material as carefully as one would an archeological dig, I did, in fact, discover exercises that were very similar to ones I had completed as a Berklee student in the early 1960s.

In 1961 I enrolled in a composition course that covered Schillinger theory. The exercises we were assigned dealt with the mathematical permutations of pitch combinations and rhythm patterns. From these exercises we learned to apply Schillinger concepts to our own compositions. One might expect that the pieces written for a basic composition course would lean toward the music of Bach, Handel, or Mozart. Surprisingly, our pieces sounded modern. There was interesting dissonance, interval angularity, and rhythmic complexity that took each of us to a new and different level. In fact, Schillinger theory helped many of us get to musical places none of us had ever been before. I still occasionally use some of Schillinger's concepts in my own writing to derive unusual scales, voicings, and rhythms.

The first two notebooks I looked at contain material on Schillinger's theory of melody. In notebook one, there is a graphic representation of the melody from Beethoven's "Minuet in G" (see example 1), which is designed to demonstrate relative pitch and rhythm placement in this well-known classic. There are also graphs of the old standards "Smoke Gets in Your Eyes," "Thanks for the Memories," and "Always and Always." These graphs illustrate tessitura, rhythmic duration, and melodic direction as visual (as well as aural) musical phenomena. We didn't use graphs in the course that I took, but I do recall seeing a graph painted onto the blackboard in our classroom in the old Newbury Street building.

Another section of book one deals with the concept of "pitch scales" (see example 2). There are one-unit "scales" that contain no intervals (in other words, one note repeated), two-unit scales with one interval, three-unit scales with two intervals, and so on up to seven-unit scales, including the familiar major and minor scales, the modes, Neapolitan minor, Hungarian, Persian, etc. This demonstrates that both familiar and unfamiliar scales can be created simply by permuting half-step combinations. For example, the major scale can be represented as 2+2+1+2+2+2+1. The altered dominant scale can be represented as 1+2+1+2+2+2+2. The symmetric diminished scale can be represented by 1+2+1+2+1+2+1+2 (see example 3). Any scale can be represented in this way. We constructed unusual scales in class using this method and then derived short pieces from them.

Notebook two explores modulation as a melodic as well as a harmonic event. The material shows how to develop tonic systems that divide the octave by equal intervals. For example, a three-tonic system in major thirds (G, B, E-flat) can create an interesting modulation scenario. That three-tonic system, for example, forms the basis for analyzing the three key centers found in John Coltrane's tune "Giant Steps" (see example 4).

Notebook three contains information on harmonic theory. There are mathematical representations of all the possible combinations of two-, three-, and four-part structures. Also included is the concept of the voice-leading "circle" that produces motion between chords in a systematic manner (see example 5). Ascending and descending root motion patterns are also described in mathematical (and therefore permutable) terms. Not only is the familiar cycle 5 (root motion in fifths) explored, but cycles 2, 3, 4, 6, and 7 are also detailed.

In volumes four through seven, additional harmonic considerations are shown and exhaustively examined in numerous musical exercises. The material points out that the familiar Western European system of diatonic harmony is just the tip of the iceberg since, according to Schillinger, any scale can form the basis of a "diatonic" or "symmetric" system from which characteristic intervals and chords may be derived. He taught that any chord could follow any other chord as long as basic voice-leading principles were observed to ensure a musical and performable result.

Other exercises cover seventh, ninth, eleventh, and thirteenth chords. One specific harmonic concept that caught my eye in notebook six was Berk's application of Schillinger's technique for voice-leading upper structures (see example 6). This relates directly to what we currently refer to as hybrids and upper-structure triads in Berklee's current curriculum.

Notebook eight deals with the rhythmic subdivision of the bar using mathematical combinations to produce various rhythms. Using "1" for an eighth note (half a beat), formulas like 5(+3) become:

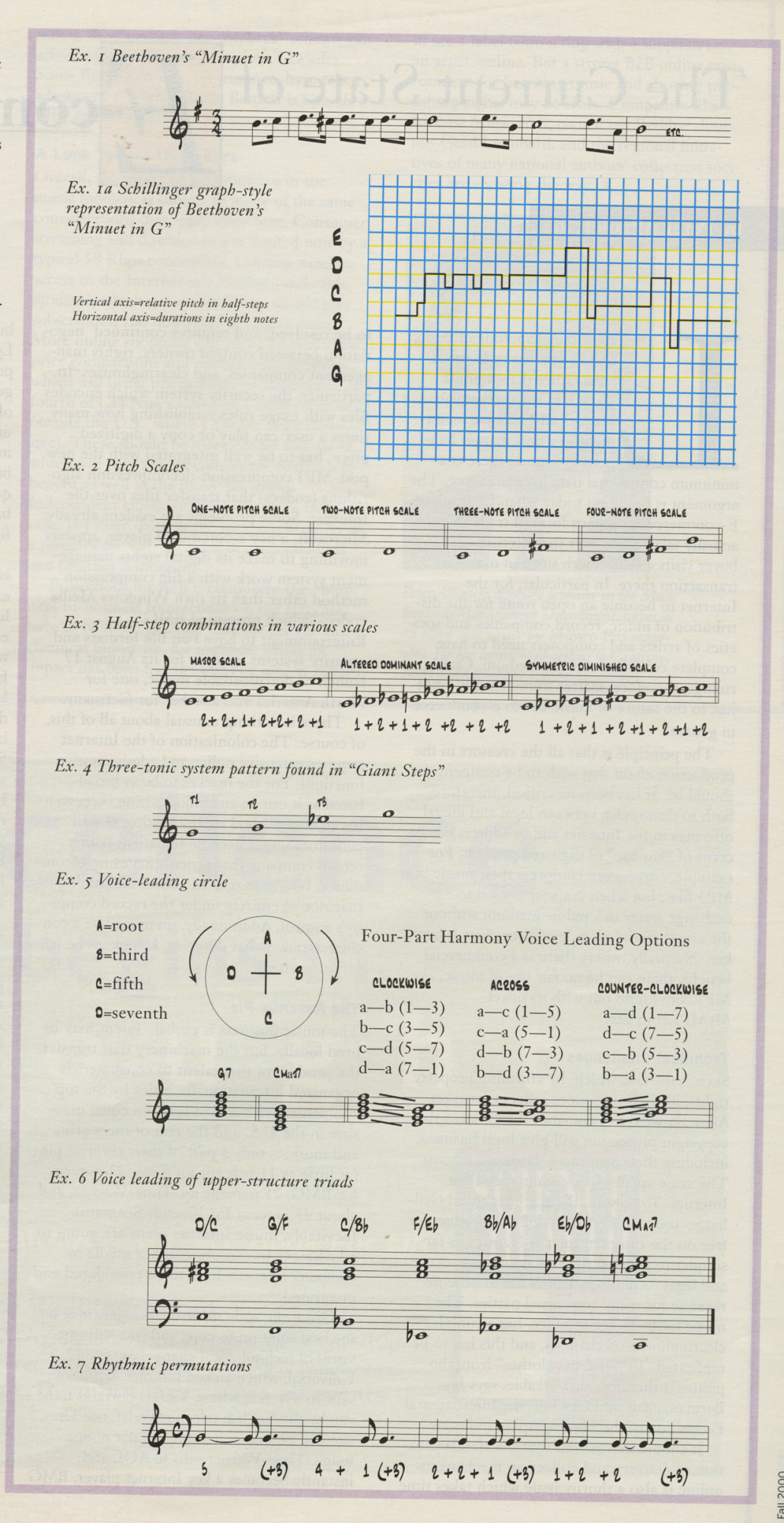
Then the 5 (or the 3) can be subdivided further, e.g., 4+1(+3), 2+2+1(+3), 1+2+2(+3) and so on (see example 7). The numbers can also stand for rests. The resulting exercises can be adapted for ear training classes to aid students learning to read syncopated rhythms. These rhythms can be turned around (3+5 instead of 5+3). Two or more lines can be written to produce rhythmic counterpoint. Pitches can be set to the various rhythms to form melodies. I remember doing these exercises in an ear training class with venerable faculty member George Brambilla. We had many pages of permutations. Notebook eight also contains more esoteric rhythm concepts that I never studied, including topics like "rhythmic resultants with fractioning."

Notebook nine contains more material on harmony and rhythm similar to that found in notebooks seven and eight. There are also a few pages on basic counterpoint, including the concept of rhythmic imitation from line to line.

Notebook 10 is about counterpoint exclusively. It addresses topics such as density and tension, resolution of dissonance, imitation, and modulation. It concludes with a discussion of how to write a fugue.

Notebook 11 contains a mimeographed course outline for a basic arranging class. I am quite sure that this was used by arranging instructors at Berklee in the late 1940s and early 1950s. It covers three- and four-part writing, open voicings, writing for saxes, brass, strings, and rhythm section, intros, and modulations. These are essentially the same topics covered in my first arranging course at Berklee.

Perusing Lawrence Berk's notebooks provided me with some interesting insights into the evolution of Berklee's curriculum and pointed to parallels between Berk and Schillinger. Schillinger took an unorthodox mathematical approach to the organization of musical elements. Similarly, Berk took an unorthodox approach to music education by systematically organizing the rudiments of jazz and other forms of modern music. By combining the knowledge he had gained as a professional musician with Schillinger's progressive theories, Berk established a successful archetype for twentieth century music education, e.g., practical career training in contemporary music. Perhaps one day people will view Schillinger's influence on Lawrence Berk as one of his most enduring contributions to American music.